A Different(ial) Approach to Memes
Introduction
A ‘meme’, as coined by Richard Dawkins, is “a unit of cultural transmission” – an idea, behaviour, or style that spreads from person to person within a culture.[3] Cultural ideas, symbols and practices that are passed down through generations would mutate due to the environment of the culture at that point in time.
In today’s context, a ‘meme’ is a virally-transmitted cultural symbol or social idea.[4] It is a concept, catch phrase or a piece of media that starts becoming more prevalent, often as a mimicry. It is propagated especially by the use of social media sites and its sharing functions (eg. on Facebook ‘share’, Twitter ‘retweet’) travelling from person to person quickly through social media.

A still from the Harlem Shake video
An example of a meme would be the Harlem shake, which is a meme in video form where a group of people perform a comedy sketch to the song “Harlem Shake”. The video would start out with one person dancing to the song alone and after 15 seconds, a group of people would join in doing crazy convulsive dance moves for the rest of the video.[5]
Harlem Shake on Google Trends
Memes and Differential Equations
There is an incentive to model memes in terms of a time-varying mathematical equation from a marketing standpoint. If we can express the popularity of a meme as a function of time, the behaviour of a topic that goes viral can be predicted. Thus, when crafting memes to market a product, advertising agencies will be able to gauge the trend of the meme’s evolution and tweak their marketing strategies in order to increase the duration of its popularity.
One limitation to any attempt to model memes is the (thus far) unpredictability of the internet. Any attempt to predict how popular a meme will be – the numerical value of its peak popularity – would fall short. However, it might be possible to study the pattern of growth and decay of a meme, which would still be very useful for those who wishes their message to linger around in the consciousness of the hive mind that is internet culture.
A differential equation is an equation that expresses the unknown function of some variable and its derivative. Effectively, it shows the relation between the quantity of some object of interest, and its rate of change.[6]
Following from its definition, memes can be thought of as the passing down of some kind of information through various social networks. Here, it can be easy to see how the rate of change comes into play with the current spread of the meme.
If one person passes the meme to two people, the meme spread is increasing by 2 at that point. If these two people then pass on the meme to two people each, the meme spread is now increasing by 4, and so on and so forth.
Generally, the more the number of people who have consumed the meme, the faster the meme would spread – this is exactly the kind of scenario that a differential equation is meant to model.
Setting up the Model
To begin setting up our model, we would equate the derivative of the equation to the equation itself, multiplied by some positive constant – since we are expecting its popularity to grow with time.
However, we need to also consider that there would reach a certain saturation point when the meme becomes “old”, or “uncool” – beyond this, the meme’s popularity would start to drop. This can be seen with the Harlem Shake example, and every other meme to come. Its decrease in popularity should follow a similar pattern of its initial increase, although with a different rate of change – thus we would expect a different negative constant after the peak occurs.
To put it in more formal terms, we can use $m$ to represent the relative meme popularity – here we are not concerned with the absolute popularity of the meme (how many people actually shared/are actively sharing the meme), but how popular the meme is compared to its peak popularity at any point in time. Thus
From here, there are two time intervals we can separately investigate: before it reaches its saturation point, and afterwards. Together with the ideas mentioned above, we can thus model the rate of change of a meme’s popularity with regards to its current popularity in a piecewise function:
Where:
Expected Properties of the Final Equation
Before solving the differential solution, it would make it clearer to list certain properties the solutions must have as a result how we have modeled the situation, or as an implication of its behaviour.
-
Since we have defined -
Since the function of a meme’s relative popularity is a real measure of a continuous trend, it must follow that the model must also be continuous at every point. Being a piecewise function, there is a possibility of discontinuity at -
If we go back a long time, there would be a point in which the memes must have been created (or if one goes back even longer, the internet would not even exist).
Thus,
It would also make sense for the same thing to happen as
Solution of Differential Equation
We can solve our differential equation by using the separation of variables technique:
Analysis of the Solution
First, we consider the properties that we previously set out that our model must have.
-
As -
The condition used for eliminating the constants have already established that -
To get a sense of how well our equation models the situation, using
This gives a graph that looks similar to the Google trend of Harlem Shake. To allow for a more direct comparison, we can redefine the time
Here on out, we can compare our model with the actual trend of the memes included.
Applying the Model
Harlem Shake
Revisiting this case with our updated model, we get the following fit:
The parameters for the fit reflects the characteristics of the meme. The basic format of a “harlem shake” is quite straightforward – begin with a lone dancer with everyone else ignoring them, and when the music drops, jump cut to everyone going crazy; this lends to itself its replicative and mutative ease. Anyone can copy these steps, and anyone can put their own spin to it – from entire campuses[8] to members of the armed forces[9] – which greatly delayed the meme’s decay.
In the analysis of this meme, we would like to introduce another basis for comparison, the decay-virality ratio. This is simply the decay rate divided by the virality. Because different memes will have different virality and decay rates, this ratio provides us with a standard basis around which we can compare how quickly or slowly it decays as compared to how quickly it grew. As we will see later, the virality of most memes vary very little from one another (of course, exceptions exists). Thus, when comparing decay rates, it makes more sense to compare this ratio, rather the decay rate directly.
Mannequin Challenge

A still from a Mannequin Challenge video by American professional basketball team Cleveland Cavaliers, featuring then First Lady Michelle Obama and LeBron James, with over 1 million views on YouTube[10]
The Mannequin Challenge is a viral video trend where people imitate mannequins, remaining frozen as a camera moves around and films them. Background music is often used in these videos, usually “Black Beatles” by Rae Sremmurd.
This trend is believed to have started by a group of students in Jacksonville, Florida in October 2016. Since then, the challenge gained popularity in high schools in other states and has been attempted by celebrities and people all over the world.
This is another meme similar in spirit with the Harlem shake, also having a simple format to follow and a small room for variety. The different backgrounds of people and environments where this meme is held add to its freshness, and its presentation as a “challenge” drives people to take up the challenge. This time, we see a steeper growth and decay rate than the Harlem shake, although the decay-virality ratio is still around the same value.
Ice Bucket Challenge

Mass participants of the ice bucket challenge
The ALS Ice Bucket Challenge was an attempt to raise awareness and fund raise for a motor neurone disease known as Amyotrophic Lateral Sclerosis (ALS). It was started by a former baseball player, Pete Frates, who was diagnosed with ALS in 2011. The Ice Bucket Challenge involves participants being filmed while a bucket of ice water is dumped over their heads. Participants can also “nominate” others on social media platforms to take up the challenge. If the challenge is incomplete by the end of the day, a hundred dollars must be donated to the ALS Association.[11]
This meme caught the attention of many celebrities and organisations, who helped to increase awareness due to their influence on social media platforms. Many have donated even after completing the Ice Bucket Challenge within twenty-four hours, fulfilling the aim of the challenge, raising over $15.6 million in donations.
The ice-bucket challenge is similar to both the previous cases in its reproducibility, but makes for an interesting case study because of high decay rate, partially due to the inflexible nature of the challenge (there are only so many ways to pour a bucket of ice over your head).
In actual fact, this meme was not designed to last – it was intended to last for only twenty-four hours, which was enough for ALS to raise the millions of dollars that they did. So a low decay-virality ratio does not need to be the end goal of a marketing strategy – memes with a high decay rate can intentionally designed and utilised optimally as the ice bucket challenge have shown.
Damn Daniel

Daniel Lara, star of the “Damn Daniel!” videos
This 2016 internet sensation was started by Joshua Holz, a student from Riverside Polytechnic High School, who recorded videos of his friend, Daniel Lara during school hours. He would do so whenever Lara wore a pair of white Vans shoes, exclaiming “Damn Daniel! Back at it again with the white Vans!” in a funny voice.
These videos were uploaded to his personal Snapchat (a social media platform) account, whereupon his peers encouraged him to “keep the videos coming”. The short videos were compiled, and the addictive catch phrase had over 45 million views in nine days.[12]
Here we begin to encounter memes of a different characteristic from the above, mainly those that are less reproducible. Unlike the previous memes, there are no challenges for consumers of “Damn Daniel!” to partake in, or for them to replicate into a form that is their own. Namely, “Damn Daniel!” is a one-way meme, of which there are only producers (the creator of the meme), and consumers (everyone else). This increases the decay rate since the source of meme production is much less than a multiple-producer model (in the previous cases, where anyone can replicate the meme in question).
Yet at the same time, the ease of access to “Damn Daniel!” is that uncomparable to the previous memes. Its medium of transmission, Snapchat, allows for videos of a length only up to 10 seconds long. This reduces the time needed to consume the meme, increasing both the likeliness of someone watching it, and the likeliness of them passing it on to someone else (one would be more willing to share something if they know that they are not taking up too much of someone else’s time). This increases the virality of the meme, in effect keeping the decay-virality challenge just a little higher than the previous memes (apart from the ice-bucket challenge).
Caveman Spongebob

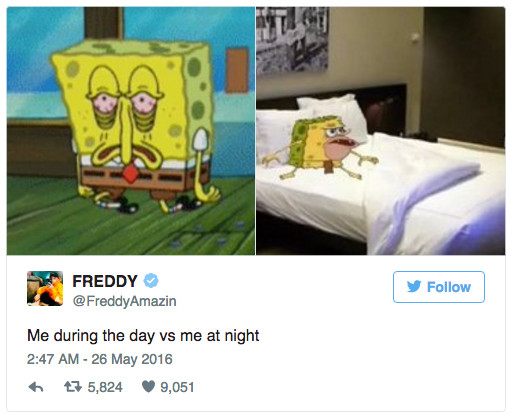
The character ‘Caveman Spongebob’ as it appears in various memes
Caveman Spongebob, also known as Primitive Spongebob, is the name given to Spongebob Squarepants’ ancestors in the animated television series Spongebob Squarepants. Images of Caveman Spongebob with an angry expression have been used as reaction images for agitating situations. Caveman Spongebob is also often used as a juxtaposition to other images of Spongebob to show contrast between ‘innocent’ behaviour and ‘wild’ behaviour.[13]
Caveman Spongebob represents another class of memes, which can be broadly called “reactions”. These are pictures that can be used in a conversation, usually as a humorous reaction. Its utility in communication allows for its constant usages, keeping the decay factor small even though it is not “replicable” in the same sense as the challenges are.
An additional aspect of the Caveman Spongebob is its relatability – as shown in the figures, the Caveman Spongebob meme can be altered to reflect everyday experiences in an amusing manner. This further influences the decay factor, keeping the decay-virality ratio low (though still higher than the challenges).
White Guy Blinking

Drew Scanlon blinking
Drew Scanlon, video producer and editor of the video game site Giant Bomb, was one of the four gamers observing his compatriot play a computer game. When heard a gamer’s comment “Farming… with my hoe here”, he shook his head with disbelief and shock.[14] The video capture of his reaction was converted into an animated Graphic Interchange Format (GIF) image, which eventually went viral as social media users adopted it to describe unexpected scenarios, or as a reaction of “say what?” or “excuse me?” in a polite way, while maintaining their disapproval at the situation.
This is also a another meme of the “reaction” class, and its characteristic is best described in contrast to the previous one. Unlike Caveman Spongebob, there is very little in terms of relatability or mutability for the “White Guy Blinking” meme – it cannot be modified or varied in any way, and has to be used as-is. This limits its use cases, increasing the decay factor. With nothing to offset this increase, the decay-virality ratio greatly increases.
PPAP (Pen-Pineapple-Apple-Pen)

A still of Japanese comedian Kazuhiko Kosaka doing the PPAP dance
Pen-Pineapple-Apple-Pen (PPAP) is a single released by Piko-Taro and played by Japanese comedian Kazuhiko Kosaka.[15] It has garnered over 110 million views on YouTube since it became viral on 25 August 2016.[16] Many social media users have also parodied PPAP, replacing the pineapple and the pen with various objects of general, and specialist, interest.
The characteristics of both the “challenge” type meme and “reaction” type meme cumulates with the PPAP.
Firstly, it is easily replicable. The dance involves only a few simple steps, and the song features the same line of lyrics repeated multiple times.
Secondly, it is also highly mutable. The meme involves two subjects, a pineapple and a pen, and a single action, that of combining one with another. To create variations on the format, one would simply need to replace the subjects with other objects, or create a subversion by involving an unexpected action.
Thirdly, as a result of the nature of its mutability, this in turn adds to its relatability. When a person modifies PPAP into a subject of niche humour, others of the same community would also find it relatable, thus keeping the meme alive.
PPAP is indeed a demonstration of the principles as set out previously, reflected not only by its low decay rate, but also its exceptionally small decay-virality factor.
Kermit

Original meme (2014)

Resurfaced version (2016)
Kermit the frog is a muppet character that is well known for its character in the Muppet’s show and Sesame street. In 2014, a Lipton advertisement aired which was named “Be more Kermit” with the notation of “But that’s none of my business” which was to used as a postscript to an insult or disrespectful remark towards a specific individual or group.[17] An example would be, “You’ve gone to the gym for six months and still look exactly the same, but that’s none of my business.”
Kermit is an interesting study of meme mutation, where its modification caused a separate sub-species, resulting in a second peak. Curiously, the characteristics of both are very similar. To model this, we took the superposition of two equations with different values of
Both subspecies is a highly-mutable “reaction”-type meme. The first sub-species involves a standard catchphrase (“But that’s none of my business”), and a preceding setup, where any situation can be filled in by anyone. The second sub-species also follows a two-part format – this time both statements can be filled in by anyone, the one requirement being that they contrast each other – a “light” side and a “dark” side.
The similarity in both the formats, and their resulting virality and decay factors, shows how despite the difference in absolute popularity, the relative popularity of both in relation to their respective peaks results in the same growth and decay pattern.
Conclusion
Superimposition of all single-peak curves along the same time scale (“White Guy Blinking” is still a developing meme, thus it gets cut off with the given time range)
Importance of virality
The above figure shows how little the growth factor varies from one another: with the exception of “Damn Daniel!”, the virality are around the value of
Importance of decay rate
Of course, it does not always mean that a high decay rate makes a meme less effective. The chief example of this is the ice bucket challenge, where the rapid decay of the meme was put to good use by the ALS association.
Decay-virality ratio
Generally, memes in similar classes share have similar decay-virality ratios even when either parameters are different – “challenge” type memes have ratios of ~0.15, while “reaction” memes vary a lot more, on the upwards of ~0.2. This property can be taken advantage of by intentionally reducing the virality of a meme (e.g. have steadier marketing strategy, where the meme is introduced slowly but regularly), which would in turn also decrease its decay rate.
Mutability
The most important influence upon the decay rate is the mutability of a meme. Here we see memes like the Kermit memes, where its ease of adaptability into not just everyday humour, but also those of a niche community, decreases its decay ratio far below those of its same class.
Limitations
As with any attempts at modelling social behaviour, exceptions and outliers are more commonplace than in the physical world – furthermore so when we try to model something as random and arbitrary as internet memes. Notable limitations includes the model’s inability to consider “bumps” in a meme’s popularity (e.g. when a celebrity gives publicity to an otherwise dying meme, like the unevenness in the otherwise smooth trend of the mannequin challenge in November 2016 with Michelle Obama’s version), or its uncertainty in modeling current memes (like the “White Guy Blinking”). The latter makes this model a general indication of trends rather a true predictor of one – it still provides useful principles for a meme’s popularity, but greatly limited in its scope.
A better method to model this will be to use a variant of the SIR epidemiological model, where we can model consumers of memes as the infected (those who have consumed and are actively promoting the meme), susceptible (those who have not yet encountered the meme), and the recovered (those who were previous consumers of the meme but are now sick of it). These three variables form a system of differential equations, which are not solvable in general, and thus require numerical methods to solve it. Thus, possible future directions might be to model a meme’s popularity with the epidemiological model.
Another limitation is the relative dataset available from Google Trends. As it is a free service, comprehensive breakdowns of the statistics, or absolute values, which might allow us to compare the absolute popularity of certain memes over others, is not available. A possible future direction of study might be to ascertain the numerical data of meme popularity, and together with the above epidemiological model, predict more absolutely the trajectory of a meme.
References
- Weng, L., Menczer, F., & Ahn, Y. Y. (2014, May). Predicting successful memes using network and community structure. In Eighth international AAAI conference on weblogs and social media. Retrieved from https://www.aaai.org/ocs/index.php/ICWSM/ICWSM14/paper/viewPaper/8081
- Wang, W. Y., & Wen, M. (2015). I Can Has Cheezburger? A Nonparanormal Approach to Combining Textual and Visual Information for Predicting and Generating Popular Meme Descriptions. Proceedings of the 2015 Conference of the North American Chapter of the Association for Computational Linguistics: Human Language Technologies. https://doi.org/10.3115/v1/n15-1039
- Dawkins, R. (1989). The Selfish Gene. Oxford: Oxford University Press.
- Gonzalez, D. (2015). Approaches to content. In Managing Online Risk: Apps, Mobile, and Social Media Security (pp. 127-152). Butterworth-Heinemann. https://doi.org/10.1016/B978-0-12-420055-5.00006-2
- Ashton, K. (2015, February 3). Why Did Everybody Do the Harlem Shake? Retrieved April 10, 2017, from https://medium.com/cuepoint/when-everybody-did-the-harlem-shake-f20147e77eb8
- Canuto, C., & Tabacco, A. (2015). Ordinary differential equations. In Mathematical Analysis I (pp. 389-423). Switzerland: Springer International Publishing.
- Statista. (2014). Internet use by age group worldwide as of November 2014. Retrieved April 10, 2017.
- Yoho, R. (2013, February 11). Harlem Shake - West Virginia University - Downtown Campus [Video file]. Retrieved from https://www.youtube.com/watch?v=4PxNWaPoCQg
- Weid, D. (2013, February 24). Marines Harlem Shake [Video file]. Retrieved from https://www.youtube.com/watch?v=CkuGHZ7lXG0
- Knowlton, E. (2016, November 11). The Cavaliers did the ‘mannequin challenge’ with Michelle Obama at the White House. Business Insider. Retrieved April 10, 2017, from https://www.businessinsider.sg/cavaliers-michelle-obama-mannequin-challenge-2016-11
- Herper, M. (2014, August 19). Think The ALS Ice Bucket Challenge Is Stupid? You’re Wrong. Forbes. Retrieved April 10, 2017, from https://www.forbes.com/sites/matthewherper/2014/08/19/think-the-ice-bucket-challenge-is-stupid-youre-wrong
- Rogers, K. (2016, February 25). We Should Probably Have a Conversation About ‘Damn, Daniel’. The New York Times. Retrieved April 10, 2017, from https://www.nytimes.com/2016/02/26/style/damn-daniel-video-vans.html
- Bergado, G. (2016, June 1). ‘SpongeGar’ memes bring out the primal in everyone. The Daily Dot. Retrieved April 10, 2017, from https://www.dailydot.com/unclick/spongegar-meme-primitive-sponge
- Cresci, E. (2017, February 23). Meet the man behind the ‘white guy blinking’ meme. The Guardian. Retrieved April 10, 2017, from https://www.theguardian.com/technology/2017/feb/23/meet-the-man-behind-the-white-guy-blinking-meme-drew-scanlon
- Chen, H. (2016, September 27). How a ‘Pen-Pineapple-Apple-Pen’ earworm took over the internet. BBC. Retrieved April 10, 2017, from https://www.bbc.com/news/world-asia-37480920
- Pikotaro Official Channel. (2016, August 25). Pen-Pineapple-Apple-Pen/PIKO-TARO [Video file]. Retrieved from https://www.youtube.com/watch?v=0E00Zuayv9Q
- Bergado, G. (2016, June 21). ‘Good Morning America’ loses all credibility by calling Kermit meme ‘#tealizard’. The Daily Dot. Retrieved April 10, 2017, from https://www.dailydot.com/unclick/good-morning-america-kermit-sipping-tea-lizard